Solutions for
Radioactivity: Carbon Dating
Q1. The carbon content of living trees includes a small proportion of carbon-14, which is a radioactive isotope. After a tree dies, the proportion of carbon-14 in it decreases due to radioactive decay.
(a)
(i) The half-life of carbon-14 is 5740 years.
Calculate the radioactive decay constant in yr−1 of carbon-14.
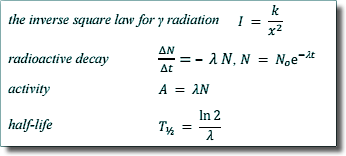
λ = ln 2/Thalf
λ = ln 2/5740
λ = 1.21 x 10-4 y-1 
(1 mark)
(ii) A piece of wood taken from an axe handle found on an archaeological site has 0.375 times as many carbon-14 atoms as an equal mass of living wood.
Calculate the age of the axe handle in years.
Let the age of the wood be t years
Nt = 0.375No
Nt = Noe-λ
t
0.375 No = Noe-λ
t
ln 0.375 = -λ
t
t = -ln 0.375/ 1.21 x 10-4
t = 8106
The age of the axe handle is 8110 years 
(3 marks)
(b) Suggest why the method of carbon dating is likely to be unreliable if a sample is:
(i) less than 200 years old,
It is difficult to measure accurately the small change in activity that would occur in only 200 years. 
(ii) more than 60 000 years old.
The activity of the sample would be very small / comparable to the background
or the ratio of C-14 to C-12 is too small
or there are too few C-14 atoms
or there is very little decay
or the level of C-14 (in the biosphere) is uncertain (this long ago) 
(2 marks)
(Total 6 marks)







