Topic Menu

Cyber-chess a chess instruction site

Inverse Square Law Experiment

Why does the world get dark so quickly as you move away from the circle of light from a campfire?

We all know that a light, such as a candle or a streetlight, looks dimmer the farther away from it we get.
The question of how much dimmer it looks was answered a long time ago. Here's a simple experiment you can perform to repeat that discovery.

x

Requirements:

    • A flashlight: A point source of light is required for this so a tiny flashlight bulb is best
    • Cardboard
    • Graph paper
    • A file card.
    • A knife or scissors.

Unscrew the front reflector assembly of the torch to expose the bulb. The bulb on the torch will come on and stay on even when the reflector assembly is removed.

c

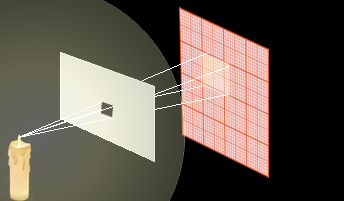
Now cut a l/2 x 1/2 inch (1.3 x 1.3 cm) square hole in the file card. Hold or mount the card 1 inch (2.5 cm) in front of the light source. The square of light made when the light shines through this hole will shine on the graph paper. Keep the distance between the bulb and the card with the square hole constant at 1 inch (2.5 cm). Put the graph paper at different distances from the bulb, and count how many squares on the graph paper are lit at each distance. The results will be easier to understand if you make a table of "number of squares lit" versus "distance." Be sure to measure the distance from the bulb.

v

The light from the bulb spreads out equally in all directions.

As the distance from the bulb to the graph paper increases, the same amount of light spreads over a larger and larger area, and the light reaching each square becomes correspondingly less intense.

For example, adjust the distance from the bulb to the graph paper to 1 inch (2.5 cm). At this distance, the graph paper touches the card. A single 1/2 inch (1.3 cm) square area will be illuminated.

When the graph paper is moved 2 inches (5 cm) from the card, four 1/2 inch (1.3 cm) squares will be illuminated on the graph paper.

When the graph paper is moved 3 inches (8 cm) from the card, 9 squares will be illuminated. At 4 inches (10 cm), 16 squares will be illuminated, and so on.

The area illuminated will increase as the square of the distance.

The intensity of light is the power per area. Since the energy that comes through the hole you cut is spread out over a larger area, the intensity of light decreases. Since the area increases as the square of the distance, the intensity of the light must decrease as the inverse square of the distance. Thus, intensity follows the inverse-square law.