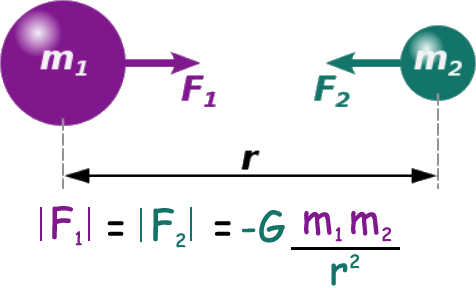Topic Menu

Cyber-chess a chess instruction site

Gravitational Force

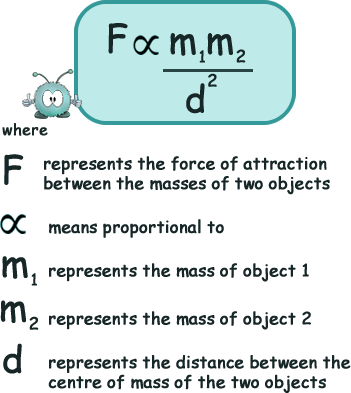
Newton's Law of Universal Gravitation describes how ALL objects attract each other's mass with a force of gravitational attraction.

Newton's law of universal gravitation states that every particle attracts every other particle in the universe with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers.

 

It is a general physical law derived from empirical observations by what Isaac Newton called 'inductive reasoning' It is a part of classical mechanics and was formulated in Newton's work Philosophiæ Naturalis Principia Mathematica ("the Principia"), first published on 5 July 1687.

When Newton presented Book 1 of the unpublished text in April 1686 to the Royal Society, Robert Hooke made a claim that Newton had obtained the inverse square law from him.

The force of gravitational attraction was found experimentally to be directly proportional to the masses of each of the objects and inversely proportional to the square of the distance that separates their centers.

 

To make this into an equation we need to add a 'constant of proportionality'.

This is 'G' - the gravitational constant. The precise value of G was determined experimentally by Henry Cavendish in 1798, almost a century after Newton's death. 

It has a value of 6.673 x 10-11 N m2 kg-2, and you will find it on your data sheet in examinations.

It is also known as the universal gravitational constant, Newton's constant,

and colloquially as 'Big G'.

The distance between the masses is denoted by 'r'

F1 and F2 are opposite in sign

File:NewtonsLawOfUniversalGravitation.svg

Mass m1 is pulling mass m2, and mass m2 is pulling mass m1... newton's third law!

The magnitude of the two forces is the same - but they pull in opposite directions.

The negative sign before the G indicates that the force of gravity is allways attractive. Attractive forces get a negative sign (think of the force between opposite charges - the product of those charges will always be negative if they are opposites and attractive....).

 

Click here to find out about 'g' - the gravitational field strength

Click here to find out about gravitational potential