Magnetic Fields
When placed in a magnetic field, fixed magnets (magnetic dipoles) tend to align their axes to be parallel with the magnetic field. That is why iron filings line up to show the field lines when sprinkled around a magnet. They become little tiny magnets are line up - axes parallel to the field lines from the permanent magnet. A changing magnetic field can induce an electric field - electromagnetic induction. Magnetic Fields are formed around moving charges (and therefore around current carrying wires - as they have a net movement of charge!). The field lines show the direction a plotting compass would point if placed at that point in the field. They always fowm complete loops (unless they start and finish on a magnet - then the domains inside the magnetic material would complete the loop) and they NEVER cross. Magnetic fields have an effect on moving charges at right angles to them - and only moving charges produce magnetic fields. If the charge you are dealing with is NOT at right angles to the field you will have to find out the velocity vector that is at right angles to the field (see module 2 of the AS course).
You have to be careful you know what you are doing with this though! The thumb points in the direction that the charged particle will experience a force towards. The forefinger points in the direction of the field. The second finger points in the direction of conventional current (points in the direction the charged particle is travelling IF IT IS POSITIVE!!). If you are looking at the effect on an electron you have to have the second finger pointing in the opposite direction to its velocity... F = qvB where
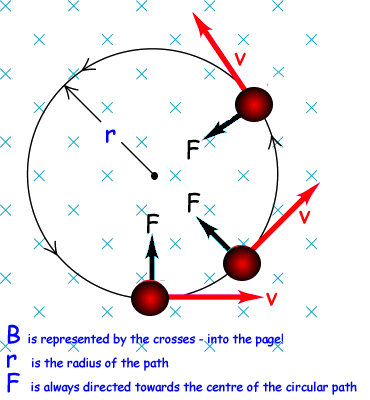
If a charge enters a uniform field it will therefore experience a constant force at right angles to its velocity - making it move in a circle.
F (magnetic) becomes F (centripetal) So qvB = mv2/r Rearranging we get that r = (mv)/(qB) = p/(qB) This means that:
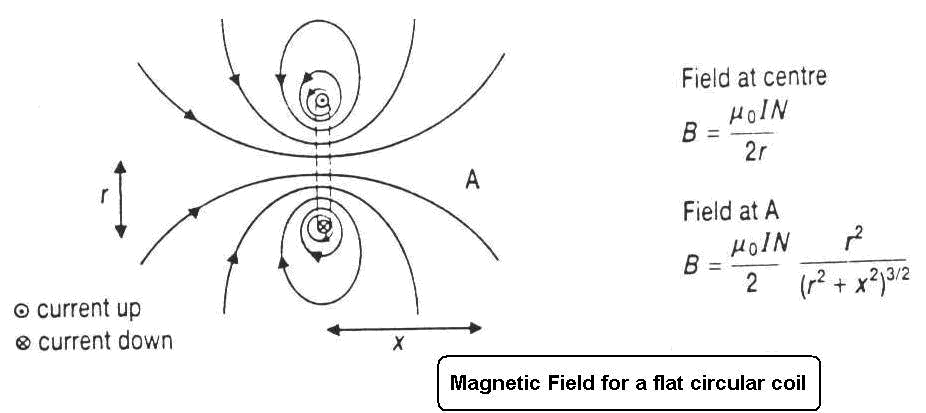
Click here to enlarge image Close to the wire the circles of the field lines are virtually uniformly spaced but they do get further apart when you get further away...
Click here to enlarge image
Click here to enlarge image
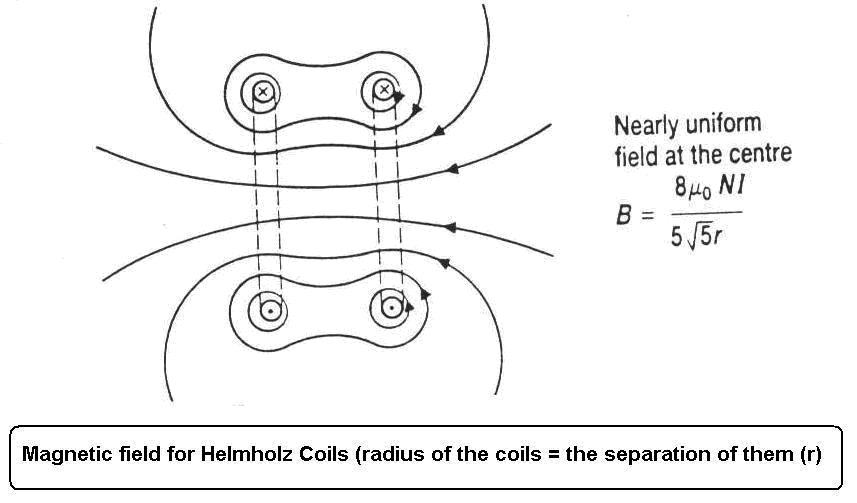
Solenoids are useful to the physicist as you can perform experiments within a uniform magnetic field and know it's value too by setting the current to give you the field you require.
Click here to enlarge image LOJ (January 2003) |
Follow me...
|

 Magnetic fields surround and are created by electric currents, magnetic dipoles, and changing electric fields. A magnetic field is a vector field that permeates space. It can exert a magnetic force on moving electric charges and on magnetic dipoles (such as permanent magnets).
Magnetic fields surround and are created by electric currents, magnetic dipoles, and changing electric fields. A magnetic field is a vector field that permeates space. It can exert a magnetic force on moving electric charges and on magnetic dipoles (such as permanent magnets).  The force the charge
experiences is at right angles to its velocity (i.e. in another plane)
and at right angles to the field lines (the three are mutually at right
angles to each other) ... you use Flemming's Left Hand Motor Rule to find
out how the charge is affected by a field.
The force the charge
experiences is at right angles to its velocity (i.e. in another plane)
and at right angles to the field lines (the three are mutually at right
angles to each other) ... you use Flemming's Left Hand Motor Rule to find
out how the charge is affected by a field.