Factors that affect the resistance of a wire

 

The resistance of an object is a measure of the how reluctant current is to flow through that object. It is given the symbol R and has the unit W (which is a greek letter omega and pronounced 'ohm') Current only flows through a component of a circuit if a potential difference (voltage) is put across it. The bigger the potential difference across its ends the bigger the current flow. This is because there is a steeper 'electric slope' in place to make the charges slide down... steeper slope - faster slide! 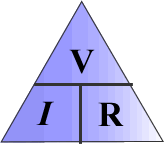The equation below is used to work out the resistance of a component from measurements of the current flowing through it and the potential difference across its ends.

 

Can you sketch a circuit diagram of a suitable circuit you could use to find out such information?

You should be able to!

V = I R Where V = potential difference in volts (V) I = current in amps (A) and R = resistance in ohms ()

 

Current is the rate of flow of charge. The charge flowing in a wire is carried by the electrons that orbit the atoms that make up the wire. As soon as a potential difference is applied the electrons generally drift in one direction (you can think of it as rolling down a slope). The bigger the p.d. the faster their average speed and the more charge will move past a point in a second - bigger current!

Increasing the number of electrons experiencing 'the slope' will mean more flow past a point in a second and so more charge passes a point in a second and more current flows.

There are four factors that affect the resistance of a wire:

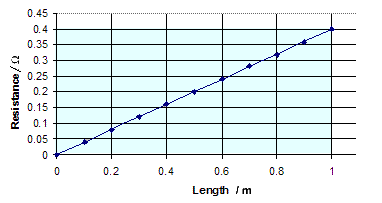
Resistance is proportional to length. If you take a wire of different lengths and give each a particular potential difference across its ends. The longer the wire the less volts each centimetre of it will get. This means that the 'electric slope' that makes the electrons move gets less steep as the wire gets longer, and the average drift velocity of electrons decreases. The correct term for this 'electric slope' is the potential gradient. A smaller potential gradient (less volts per metre) means current decreases with increased length and resistance increases.

Resistance is inversely proportional to cross-sectional-area. The bigger the cross sectional area of the wire the greater the number of electrons that experience the 'electric slope' from the potenetial difference. As the length of the wire does not change each cm still gets the same number of volts across it - the potential gradient does not change and so the average drift velocity of individual elecrons does not change. Although they do not move any faster there are more of them moving so the total charge movement in a given time is greater and current flow increases. This means resistance decreases. This does not give rise to a straight line graph as cross sectional area is inversely proportional to resistance not directly proportional to it.

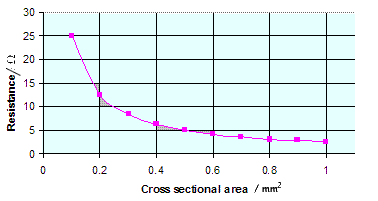
Physicists like to get straight line relationships if they can.... can you think of a way of getting a straight line graph through the origin? What would you have to plot?

Resistance depends on the material the wire is made of. The more tightly an atom holds on to its outermost electrons the harder it will be to make a current flow. The electronic configuration of an atom determines how willing the atom will be to allow an electron to leave and wander through the lattice. If a shell is almost full the atom is reluctant to let its electrons wander and the material it is in is an insulator. If the outermost shell (or sub-shell with transition metals) is less than half full then the atom is willing to let those electrons wander and the material is a conductor.

A graph for this would be a bar chart not a line graph.

Resistance increases with the temperature of the wire. The hotter wire has a larger resistance because of increased vibration of the atomic lattice. When a material gets hotter the atoms in the lattice vibrate more. This makes it difficult for the electrons to move without interaction with an atom and increases resistance. The relationship between resistance and temperature is not a simple one.

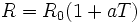
( (alpha) is the thermal resistance coefficient)

-------------------------------------------

At A level we will put these equations into an equation. Check out the page and read a little further.....